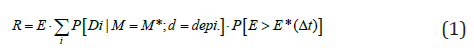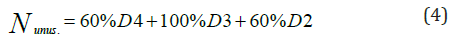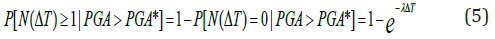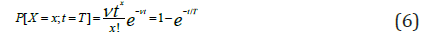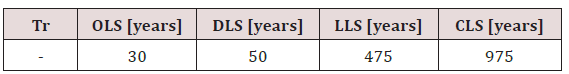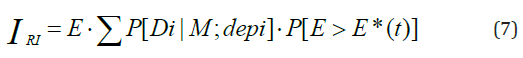Expected Seismic Risk in a District of the Sant’antimo’s Historical Centre Volume 2 - Issue 1

Antonio Formisano¹* and Nicola Chieffo²

• ¹Department of Structures for Engineering and Architecture, University of Naples “Federico II”, Italy
• ²Faculty of Architecture, Politehnica University of Timişoara, Romania

Received: April 17, 2018;   Published: April 26, 2018

Corresponding author: Antonio Formisano, Department of Structures for Engineering and Architecture, University of Naples “Federico II”, Italy

DOI: 10.32474/TCEIA.2018.02.000130

Abstract PDF

---

Abstract

The current study focuses on a sub-urban sector of the historical centre of Sant’Antimo, placed in the province of Naples (Italy), in order to estimate the expected damage scenario due to earthquakes by using a probabilistic approach. First, aiming at performing an exposure analysis, the typological and structural characterization of buildings within the urban sector has been done with the purpose to redact the CARTIS form, which was developed by the PLINIVS research centre in cooperation with the Italian Civil Protection Department in the framework of the ReLUIS research project. Subsequently, the vulnerability form developed by the first Author has been used for the vulnerability assessment of the study area. Furthermore, the typological fragility curves, according to the EMS-98 scale, have been derived for the building stocks located in the sub-urban sector. Finally, the probabilistic approach for the urban risk evaluation has been applied in order to derive the damage scenario for a set of different magnitudes and site-source distances.

Keywords: Seismic risk assessment, CARTIS form, Damage scenarios, Fragility curves, Probabilistic method

Keywords: E: Exposure, V: Vulnerability, H: Hazard, DSHA: Deterministic Seismic Hazard Analysis, DPM: Damage Probability Matrices, UNDRO: United Nations Disaster Relief Co-ordinator

Literature Review

The large-scale seismic risk is a useful tool for assessing the susceptibility of a sample of buildings to overcome, in a given period of time, a certain seismic event of an assigned entity. Seismic risk can be understood as the combination of three factors, such as Exposure (E), Vulnerability (V) and Hazard (H). The Exposure is connected to the nature, quantity and value of properties and activities of the area that can be influenced directly or indirectly by the seismic event. Vulnerability can be defined as the intrinsic potential of buildings to suffer a certain level of damage when subjected to a seismic event of defined intensity. Finally, Hazard is considered as the occurrence probability of the asymptomatic event of a certain intensity in a specific site, it depending mainly on the geographic position and the geological characteristics of the site where the event is expected [1-3]. These three factors are interconnected to each other and they are influenced by uncertainties due to the variability of the variables involved, represented by buildings in the case under study.

However, the risk, independently from the natural phenomena, is to be considered as the attainment of a level of social and economic “losses” [4]. These losses are commonly identified in terms of costs that should be supported for the system to restore its original configuration. The protection strategies of built-up areas must, therefore, represent a useful and concrete investment tool for the planning of the risk mitigation and for the protection of both people and constructive heritage. As a result, a model for vulnerability assessment and for the evaluation of economic and social losses would not only be able to estimate future potential losses due to the occurrence of earthquakes that can affect a particular region, but it should also be used to prepare and implement risk mitigation measures [5]. Generally, the heterogeneity of buildings, especially in historical centres, is still one of the most important issues concerning the large-scale seismic risk evaluation.

The methodologies used for large-scale estimations are mainly based on observational data for a significant sample of buildings considered as isolated [6], therefore neglecting the so-called building aggregates, where interactions and the connections among adjacent buildings modify significantly their seismic behaviour. Generally, the most refined analysis methods, based on the FEM technique, for evaluating these interactions among buildings placed into historical centres are generally time consuming and require greater computational efforts. So, for the evaluation of the seismic vulnerability of building aggregates, which represent the heart of the historical centres of Mediterranean area countries, speedy methods based on collection of simple data (drawings and in-situ inspections) are proposed with the purpose to provide a fast vulnerability indicator [7].

The method was initially developed by the Italian National Group for the Defence against Earthquakes (GNDT), which take profit of post-earthquake damage observations of masonry buildings in Italy [8]. Subsequently, quick methods were proposed by many European researchers into several case studies in Spain [9], Italy [10], Romania [11], Portugal [12-14] and Iran [15]. In addition, some other studies for the urban seismic vulnerability assessment, including estimation of social costs and human losses, were also delivered [16,17].

The peculiarities of these methodologies lie in the fact that they can be combined with the macroseismic method [18] for the assessment of damage scenarios. In particular, the macroseismic methodology is able to evaluate the susceptibility at damage of building stocks under variable seismic hazards usually defined according to the EMS-98 macroseismic intensity scale [19]. The possibility of identifying the most vulnerable samples of buildings allows mitigating the effects of seismic phenomena. On the other hand, the probabilistic approach for seismic risk estimation was introduced in the late 1960’s [20]. It is particularly useful for the ability to provide results that can be easily overlapped with other types of environmental risks.

The use of seismological data strictly connected to the characterization of seismogenic sources in terms of maximum expected magnitude and recurrence (number of events “E” in a period “T”) of the phenomenon have allowed to define more and more scenario analysis models accurate for earthquake mitigation. The intensity occurrence frequency, corresponding to a ground motion with a certain return period, requires a continuous observation period equal to ten times the return period, assuming that ten observations are sufficient to get a good estimation of the return frequency. Similarly, making use of the confidence interval concept [21], the number of years of continuous observation necessary to validate ground motion hazard estimates at a site is analytically derived.

An alternative to the seismic risk probabilistic evaluation method is the Deterministic Seismic Hazard Analysis (DSHA), which refers to the maximum credible event able to produce what is reasonably considered the highest level of shaking into a given site. The method takes into account the historical seismicity, the observed events and the characteristics of sources that may affect the site in order to define the design earthquake in terms of magnitude, distance and focal mechanism. The DSHA does not consider the rate of recurrence of earthquakes, but contemplates the scenarios able to generate the level of shaking probably more felt in the considered site; in contrast, the probabilistic approach takes into account all the possible shaking scenarios [22].

The seismic risk analysis on a regional scale requires the development of simplified methods and models capable of characterising both the capacity of buildings exposed to risk and the seismic demand. Therefore, a large-scale risk analysis does not refer to individual structures, but to classes of buildings having vulnerability expressed as a function of structural parameters collected by standard in situ investigations. Another interesting method for urban risk evaluation is based on the holistic approach [23], which describes seismic risk through an index. Expected building damage and losses in the infrastructure, obtained from future loss scenarios, are basic information for the evaluation of physical risk in each urban unit of analysis. Starting from these data, a physical damage index is obtained. The proposed method is developed for a multi-hazard evaluation and, therefore, it is necessary to dispose of physical damage estimations for all the significant hazards. Often, when historical information is available, the principal hazard, and thus the most potential critical situation, can be usually identified.

The risk holistic evaluation by means of indices is achieved affecting the physical risk with an impact factor obtained from contextual conditions, such as the socio-economic fragility and the lack of resilience, that aggravate the initial physical loss scenario. Available data about these conditions at urban level are necessary to apply the method. From the described scenario of available analysis methods it emerges that the choice of the most reliable one is a very critical issue, since all of them could predict well the seismic hazard into a given urban area. In the current paper, the seismic scenario analysis based on the probabilistic approach has been used. It has been applied to an urban sector located in the municipality of Sant’Antimo, a district of Naples (Italy), with the final aim to define the risk index of the analysed area starting from the recurrence of seismic events in a certain return period.

The Historical Centre of Sant’antimo

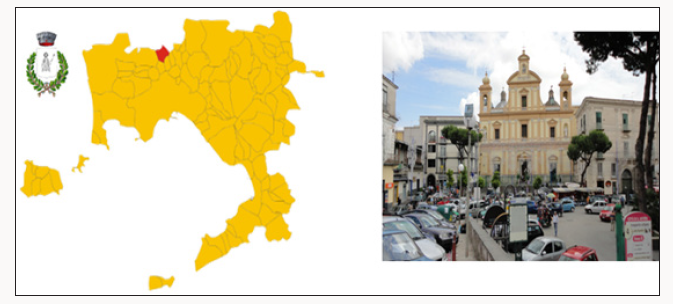
Sant’Antimo (Figure 1) is an Italian town with 33.905 inhabitants located 67m on the sea level and placed 16 Km far from the historical centre of Naples. The urban centre is developed between the Appia Street (East) and the Napoli-Foggia railway line (West), where a dense network of roads from the various centres of this area is convergent. The built-up area of Sant’Antimo appears rather compact and expands radially. From the historical and geographic point of view, it is part of the Frattamagiore’s area and constitutes an urban unicum with Melito. The first urban nucleus of Sant’Antimo dated back from IV - III century before Christ. Subsequently to the regular layout of the cadastral division, in the V century before Christ the characteristic road scheme of the medieval period, with the characteristic winding roads that crossed the agglomerations mostly erected around a church or a castle, was developed. In the case of Sant’Antimo the medieval nucleus arose in the presence of both artefacts. The period from VI century to XVI one showed the changing political conditions of the Campania Felix territory. In fact, since the year 476, with the fall of the Western Roman Empire, the period of foreign domination and political instability in the region began.

At the urban level it can be assumed that the continuous barbarian invasions for the conquest of the territory have led the population to abandon the scattered settlements and to concentrate in few more secure urban habitats, generally around the Christian places of worship. The traditional historiography of Sant’Antimo helps to support this thesis and provides information about its origins, establishing in 816 the foundation of the town, when the duke of Naples, Antemio, founded an aedicule in honour of the saint. In the middle of the town there is the main square, today called Piazza della Repubblica, where the Baronial Castle is located (Figure 2). This castle was built in the Renaissance period, as it is noticed from the characteristic architectural structures, such as the entrance hall, the loggia made of piperno and the porticoes. The central and lateral towers, instead, refer to the original medieval structure. Until the beginning of XIX century, the castle was surrounded by two gardens: one in front of the building, located near the current Republic square, the other placed behind the building, covering all the current area of Trieste and Trento Street.

The noble feudalists, who lived there, namely the Dukes Revertera until 1629, the Dukes and Princes Ruffo and the Princes Mirelli until 1806, administered the feud of Sant’Antimo, conditioning its socio-economic development. In fact, other than rents of the Feudal lands, they offered the use of own services after the payment of a tribute. In addition, they had the ability to both appoint the judge of the territory for the administration of justice and validate appointments, as well as the capacity to dismiss the elected people from the municipal administration. The town of Sant’Antimo was an agricultural centre of primary importance until the middle of XX century, when the treatment of a remnant of the processing of grapes allowed to play the industrial role of the world’s largest producer of the so-called Cremore of Tartaro, a substance which is often used in combination with small amounts of baking soda in baking powder for desserts. This work, already present in the early XVI century, assumed, until the beginning of the XX century, a fundamental function in local development and the determination of a widespread well-being, which guaranteed the civil and industrial development of the entire area. The Cremore of Tartaro, obtained for hot treatment, in large copper pots, from the residues of grapes, was then defined by the Britannica Encyclopedia as “S Antimo’s Cremore “. In this context, education spread and a class of professionals formed and distributed their experiences throughout the country: men as Nicola Romeo, founder of the Alfa Romeo company, and Cardinal Alessandro Verde, born at that time.

The Cartis Form

Large scale risk analyses at national and regional levels, with regard to natural phenomena such as seismic events, require qualitative and quantitative evaluation of the exposed elements. In this framework, the typological and structural characterization of built-up areas has usually the intent to investigate the features of constructions from the qualitative point of view. In fact, the constructive techniques were differentiated over the centuries, due to local cultures and urban conditioning, which in some cases had a major impact on the characteristics and qualities of buildings, resulting in substantial differences also in terms of response towards seismic events. The CARTIS form (acronym of Typological and Structural Characterization of Buildings) is aimed at detecting common building typologies within the communal and subcommunal areas (called sectors), characterized by homogeneity in terms of age and constructive techniques [24].

The form was initially developed by the PLINIVS research centre, in collaboration with the Italian Civil Protection Department (DPC), within the ReLUIS 2014-2016 Research Project for the evaluation of Italian territorial exposure. This form mainly refers to ordinary buildings (masonry and r.c), with regular inter-storey heights and spacing among vertical structural elements. The survey activity by the CARTIS form should be extended to the entire municipal or parts of it, thereof, provided that the “population of buildings” is exhaustive. The form is divided into four sections:

a) Section 0: for the identification of the Municipality and the sectors identified therein;

b) Section 1: for the identification of each of the predominant typologies characterizing the generic sub-sector of the assigned Municipality;

c) Section 2: for the identification of general characteristics of each typology of constructions;

d) Section 3: for the characterization of structural elements of all individuated construction typologies.

The subdivision of the territory must be addressed taking into account historical, bibliographic and documentary inquiries that allow the various constructive phases of buildings to be defined, implicitly drawing upon the indications for a final compartmentation. To this purpose, bibliographic and documentary sources can be juxtaposed by cartographic and cadastral ones. Aerial view photos and photos from Satellite, also using the most modern tools available through the web, can be also useful. In this context, the assessment of the exposure of residential properties may be proposed in terms of “inventory characterization” of the population of residential buildings. This can be obtained through the statistical evaluation of the distribution of ordinary buildings, grouped into seismic vulnerability classes, on a territorial scale with the built-up area divided into square grids having minimum side of 500 m. In the examined case study, the sub-sectors have been identified taking into account the construction period and, consequently, the normative codes used for their erection.

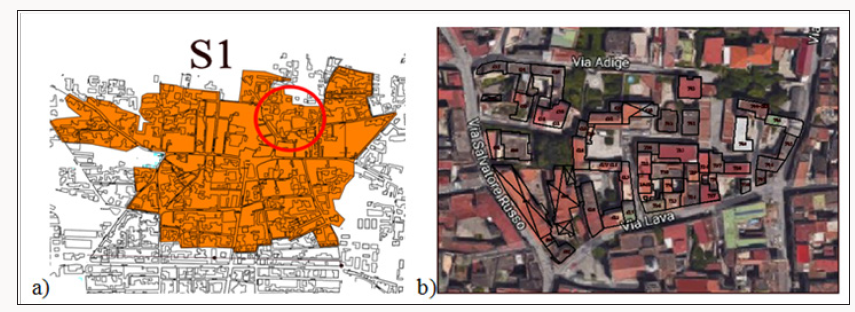
First, the first built area, characterized by buildings erected in both the early years of the last century and the post-war period, has been identified. Subsequently, the so-called expansion areas have been identified, giving rise to the definition of the remaining compartments. The use of the PRG (General Regulator Plan) cartography, dated 1977, superimposed on the present cartography of the Municipality, has allowed defining the historic centre area, characterized by almost all of masonry buildings. The buildings of the first establishment were masonry constructions made of rough stones, especially built in the first post-war period. After that period, the buildings were made of square masonry blocks. With the advent of reinforced concrete, around 1970s the first buildings were erected and grouped into construction blocks. There were also a high percentage of structures assembled in aggregates with complex geometries, especially in the area of the historic centre. For the study area, six sub-sectors have been identified (Figure 3).

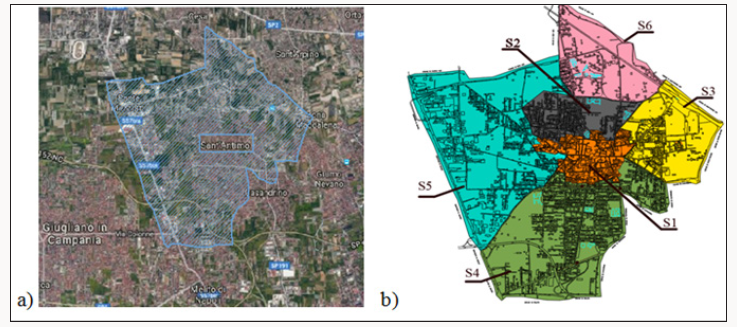
In the current paper the attention is focused on the typologicalstructural characterization of a sub-sector of the sector 1, circled in red in Figure 4. This sub-sector represents the first residential centre of the Sant’Antimo municipality, with structures built in the early 1900s and after the Second World War. This area represents the centre of commercial activities and includes also numerous historic buildings, such as the Baronial castle, having Renaissance style but with the first implant dated from the Middle Ages, the Church of Santo Spirito and the Chapel of Sant’Antimo’s priest and martyr. In that area, the buildings are erected in aggregate characterized by the lack of effective connections. The prevailing construction type is a masonry structure made of tuff block stones, which represent the local masonry obtained by extracting stones from the ground. This area is characterized by the presence of cavities with depths of more than 30m.

The masonry walls, usually with thickness of about 65cm, are of sack type with the interspace among external layers filled with stone remains and mortar. The foundations consisted in arranging the masonry walls directly on the ground at a depth of about 1.50m. In addition, there were numerous roofs without the presence of steel ties. On the other hand, albeit in small numbers, r.c. structures were also detected. These constructions were realized over the years from 2000 to 2017, often as result of the demolition of old buildings and the re-construction of new ones. Exposure analysis of an area refers to the nature, quality and quantity of risk assets. Therefore, the estimate of exposure consists on both quantification of artefacts (buildings, infrastructures, etc.) and evaluation of the number of people likely to be involved in the seismic event and able to react to it. In general, populations, artefacts and buildings are chaotically distributed in the territory: exposure analysis tends to evaluate the different concentration levels. There is a direct proportionality between the concentration of the risk elements and the exposure level. In fact, greater the risk elements at risk and greater the exposure will be.

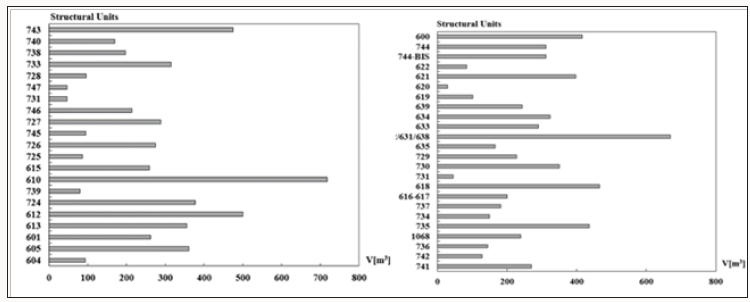
In any case, in order to make a more accurate assessment, the analysis of exposure should not be linked solely to the number of risk elements concentrated in a certain area, but it should be also associated to the evaluation of the function importance that is exerted on the territorial system. In the current study, the seismic exposure evaluation is based on the calculation of the total volume V, expressed in m3, of buildings within the inspected area (Figure 5). This choice takes into consideration the possibility of conducting an urban-scale seismic risk analysis, which is not influenced by the use of exposed buildings, but is a function of the volume of buildings. In this case, there is a possibility to solve the problem concerning the building category using the volume of such aggregates as the sole exposure indicator. As can be seen in Fig. 9, there is a marked heterogeneity of volumes, ranging from a minimum value of 28.6m3 for aggregate 620 to a maximum value of 717m3 for aggregate 632. Therefore, greater volumes correspond to greater susceptibility at exposure.

Seismic Vulnerability and Fragility Analyses

The vulnerability assessment of the sub-sector chosen within the historical centre of Sant’Antimo is considered as initial stage of a more wide study having the final purpose to evaluate the seismic risk of the whole urban built-up area investigated. The inspected masonry aggregates generally develop in elevation from 2 to 3 stories. The inter-storey height is about 3.00-4.00m for the first level and 3.00-3.50m for other floors. Horizontal structures are made of either steel-hollow tile floors or timber ones. Roofing structures are often composed of double pitch r.c. beams with clay tile covering (Figure 6). In many cases the presence of an incongruous and brittle binder in the walls, which lost over time its characteristics, compromises the static nature of the buildings themselves and, sometimes, of the whole aggregate. Therefore, renovation works to be carried out are planned, they usually leading towards the construction of new structural parts generally not perfectly linked to the existing structures.

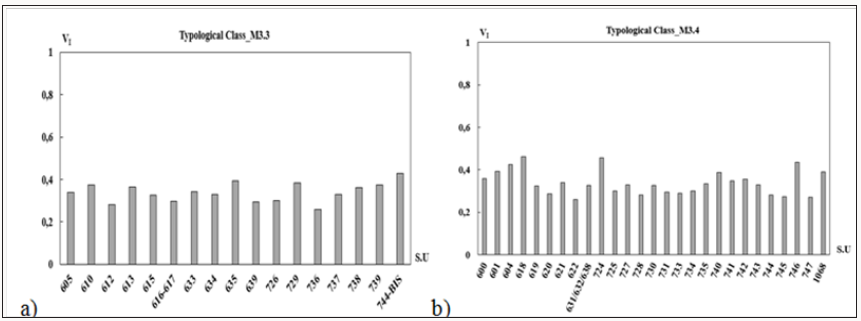
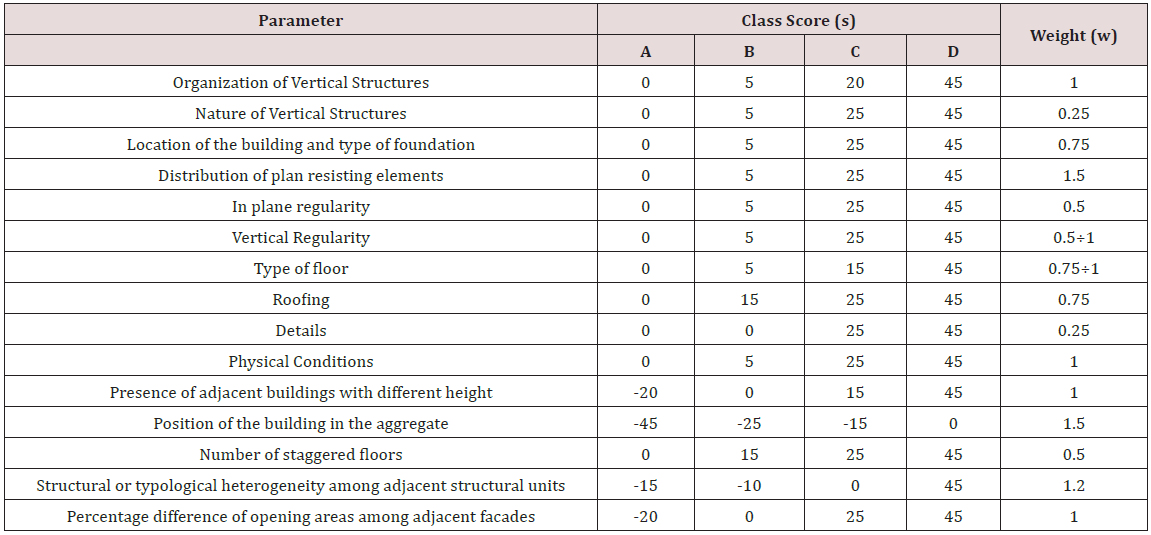
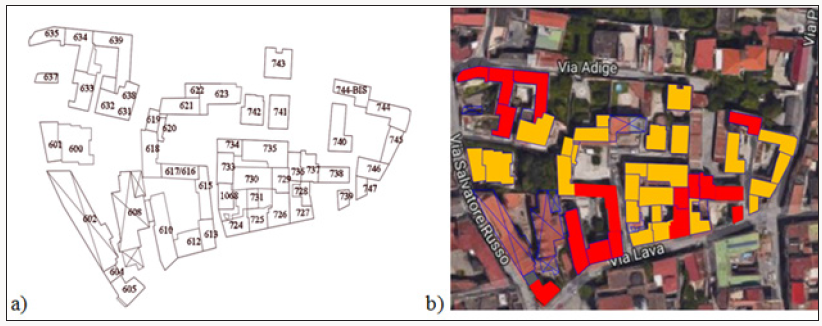
The presence of these vulnerability factors increases the possibility of collapse and instability of such historical buildings when they will be subjected to seismic actions. The lack of connections among perimeter walls orthogonal to each other (corners) does not guarantee a global behaviour of the structure. As a negative consequence, in case of earthquake, the activation of the most risky out-of-plane collapse mechanisms (overturning, vertical arch effect, horizontal arch effect, corner overturning), that may invest one or more structural units, can occur. The sub-sector under study is composed by 44 buildings, which are classified according to the Building Typology Matrix (BTM) into M3.3 class masonry structures with steel floors and rc slab (36%) and M3.4 class masonry structures with rc floors (64%) (Figure 7).

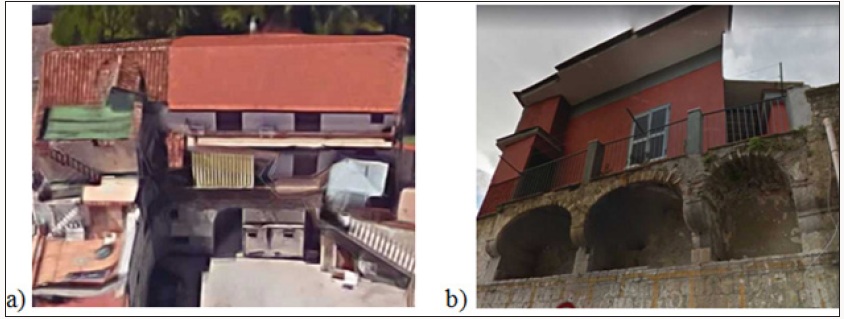
Aiming at implementing a quick seismic evaluation procedure for masonry aggregates, it has been used the new vulnerability form proposed by the first Author (Table 1) [25,26,27]. The application of such a form to the selected sub-urban sector has allowed evaluating the seismic vulnerability of investigated buildings, as illustrated in Figure 8. In particular, from previous results, it is possible to notice that the most vulnerable aggregate of the typological class M3.3 is the number 744-bis, which has a vulnerability index VI= 0.46. On the other hand, for the typological class M3.4, the aggregates having the highest vulnerability are those with numbers 618 and 724, showing indexes equal to 0.46 and 0.45, respectively. These aggregates, composed of tuff stones, have an irregular configuration, both in plan and in elevation, and they are not in a very good health state (Figure 9).

The Damage Probability Matrices (DPM) expresses the occurrence probability of a certain damage level of different typological classes for different seismic intensity levels. They can be generated by referring to a generic damage scale expressed in terms of costs (such as the ratio of the repairing cost over the reconstruction one), which can be intended either in phenomenological terms or according to a qualitative estimation of the different damage degree that buildings may undergo in case of seismic events. In the case under study, the DPM have been derived for the 2 vulnerability classes of buildings (M3.3 and M3.4) by considering macroseismic intensities from V to XII (Figure 10). From the practical point of view, DPM can be constructed after binomial coefficients are known. In the examined case, the study under consideration is that of the GNDT SSN-2002 working group [28], whose binomial coefficients are obtained from statistical analysis of the damage suffered by the various building types on the basis of occurred earthquakes.

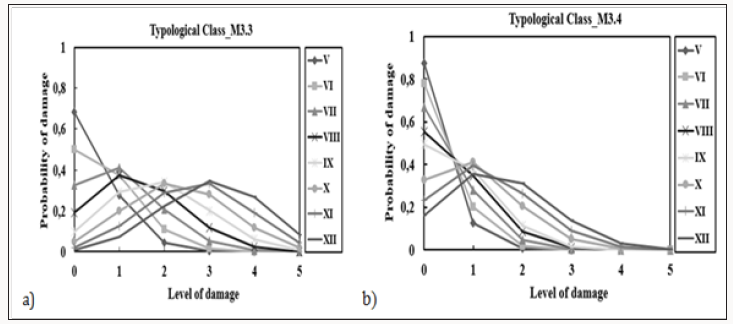
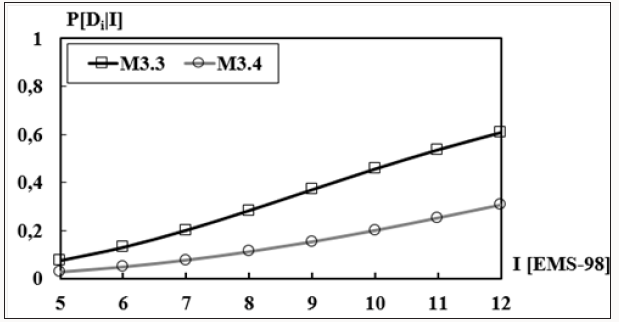
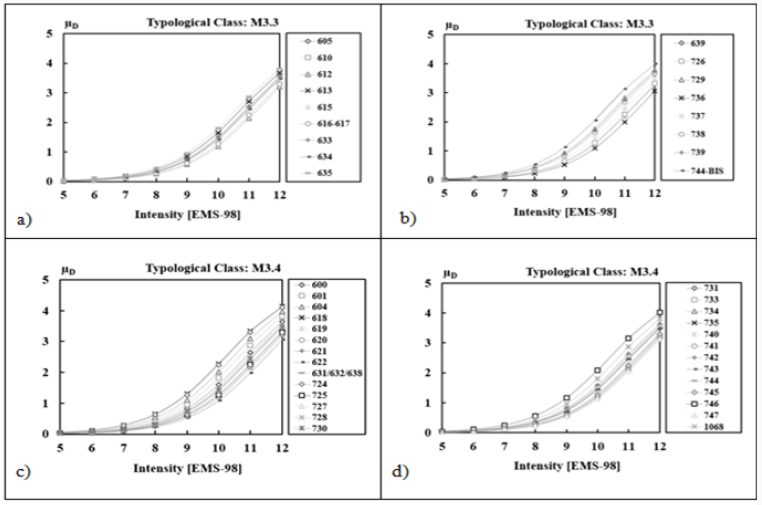
The achieved binomial distribution, corresponding to the conditional probability of having a certain damage threshold (Di) depending on the macroseismic intensity (I) (Figure 11). In this figure, the binomial distribution has the highest binomial coefficients for the M3.3 typological class, while the M3.4 one exhibits the best seismic performances with significantly reduced values of those coefficients. Subsequently, the fragility curves have been derived to estimate the collapse probability of analysed structural units under assigned seismic actions. More in detail, these curves can be properly defined as the probability P[SL|I] that a building reaches a certain limit state “LS” at a given intensity “I” defined according to the European Macroseismic Scale (EMS-98).

In particular, they depend on both the vulnerability index (IV) and the ductility factor Q, the latter variable according to the ductility of typological classes. In order to provide the best vulnerability function between the GNDT method and the EMS-98 one, a ductility factor Q equal to 2.3 has been considered. In order to assess the damages should be suffered by building aggregates under different earthquakes [29-31], the methodology proposed by Lagomarsino, Giovinazzi [32] for plotting fragility curves illustrated in Figure 12 has been used. The generated fragility curves depend on both the vulnerability index of buildings and the macroseismic intensity. Starting from the above curves, a seismic damage scenario for the examined urban sector of Sant’Antimo has been derived, as shown in the next section.

The Seismic Risk Assessment

The risk is defined for management and mitigation purposes as potential economic, social and environmental consequences of dangerous events that may occur within a given period of time. However, in the past, the concept of risk has been fragmented in many cases, according to each scientific discipline involved in its assessment. Risk requires a multidisciplinary assessment that takes into account not only the expected physical damage, the number and type of casualties and the economic losses, but also the conditions of social fragility and the lack of resistance conditions that favour second-order effects when a hazardous event hits an urban centre.

According to the United Nations Disaster Relief Co-ordinator (UNDRO), the risk R can be intended as linear combination of hazard, vulnerability and exposure as follows [33]:

Where E is the exposure, P [D_i|M=M*; d=depi] is the conditional probability to have a specific damage threshold (Di), given magnitude (M) and epicentral distance (d_epi) and P[E>E*(Δt)] is the seismic hazard, or rather the probability that a seismic event (E) with certain IM (Intensity Measure) occurs in a given time (T).

In the case under investigation, the exposure assessment is based on the analysis of individual aggregates volumes. The severity of seismic damage has been analysed thanks to predictive analyses where, during the earthquake, buildings with the same structural characteristics would be subjected to a damage that decreases when the epicentral distance increases.

To this purpose, a moment magnitude range of [4÷6] and epicentral distances varying in the range [5÷30Km] have been defined. Subsequently, the attenuation law of seismic effects according to the formula proposed by Crespellani, [34] has been taken into account as follows:

I = 6.39 +1.756Mw− 2.747ln(D + 7) (2)

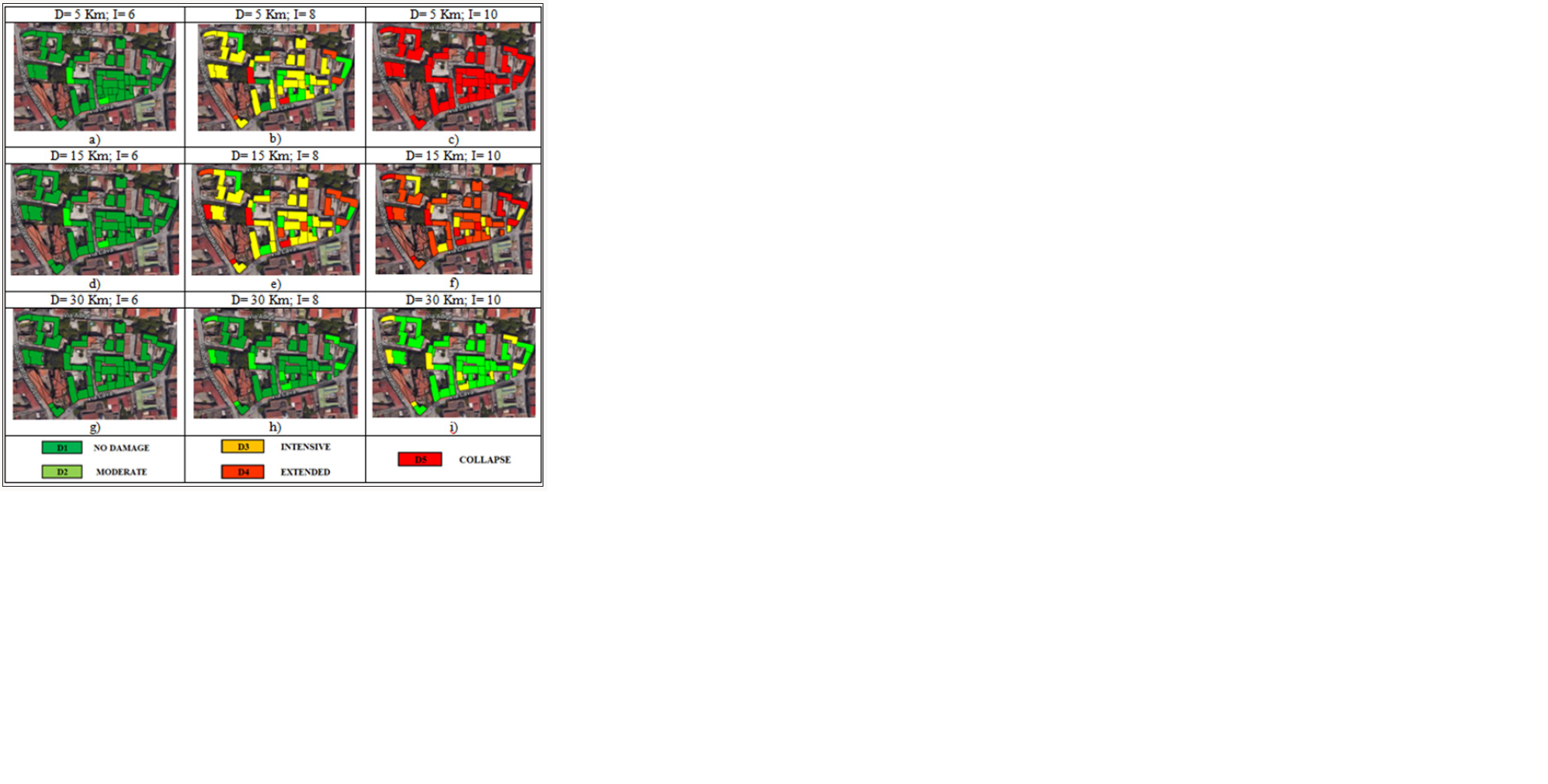
Where Mw is magnitude and D is the site-source distance expressed in Km. The correlation law between seismic intensity and moment magnitude for different epicentral distances (Figure 13). Later on, considering the damage parameter μD representative of the damage levels of the EMS-98 scale, the expected number of buildings that undergo a certain damage level under different grade earthquakes has been determined for the inspected subsector of Sant’Antimo. So, the damage maps for nine combinations of epicentral distances and seismic intensities have been plotted (Figure 14).

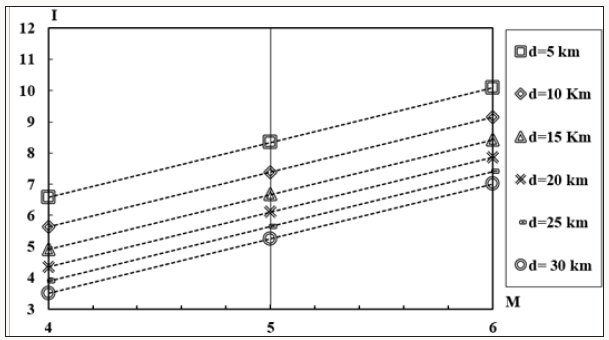
From the previous picture it is apparent that the seismic event effects depend mainly on the distance of the site from the seismogenic source. In fact, it is possible to note that, when increasing the epicentral distance, the expected damage tends to decrease because the seismic intensity attenuates much more. As an example, for an epicentral distance of 5km and a seismic intensity of 10, through the attenuation law proposed by Crespellani, the collapse of all buildings (damage level D5) should occur. Differently, by increasing the epicentral distance up to a maximum of 30 km and by leaving unaltered the magnitude, the seismic effects are mitigated with expected damage levels D2 (moderate) and D3 (intensive). In addition, for seismic intensities between 8 and 12, aggregates belonging to the M3.4 typological class show damages greater than those of M3.3 class aggregates because the former have largest geometric irregularities which tend to worsen their seismic behaviour.

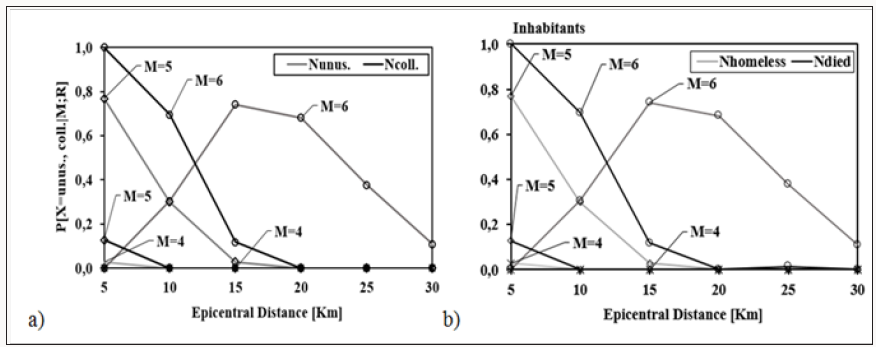
The study developed in [35] showed that the seismic damage disaggregation can be intended as a multifactorial operator that allows to evaluate the contributions of different seismic sources (d_epi) at the global damage threshold of the site (Figure 15). The scenarios obtained through the disaggregation process, therefore, allow obtaining information regarding seismic hazard by setting magnitude and epicentral distance. In particular, this process allows correlating the contribution of different scenarios induced by a magnitude (Mwi) of a given intensity (I) on the expected seismic damage (D_i). In Figure 15 it can be noted that, for a magnitude M=4 and variable epicentral distances, the most probable damage is that with threshold D1 (no damage). This means that, independently from the epicentral distance, buildings have a probability of about 100% chances to overpass the D1 damage level. On the other hand, for M=5 and for M=6, the expected major damages are characterized by levels D3 (intensive) and D5 (collapse), respectively. Based on these damage data, the number of collapsed and unusable buildings is calculated by means of the following equations [35]:

N_coll. =100%D5 + 40%D4 (3)

In Figure 16a it is perceived that, when increasing the epicentral distance (R), the number of buildings involved in the collapse tends to decrease. In fact, for R=5 Km and M=6, there is the global collapse of the whole analysed sector (overcoming probability of the D5 level equal to 100%), while for R=5Km and M=5 the probability of collapse is 13%, and the probability to have unusable buildings is 77%. The probable number of people died due to the buildings collapse and homeless inhabitants has been also assessed. The number of died and homeless depends on the average number of residents per building for each cadastral sector. In this context it should be pointed out that the maximum number of residents in the study area is equal to 153. In Figure 16b it is possible to notice that the number of people involved in the collapse is increasing with the earthquake magnitude. For magnitude M=6 and epicentral distance R=5Km, it can be noted that the probability of having all people died is equal to 100%, whereas for M=5 the probability to detect homeless and died people is equal to 77% and 12%, respectively.

Regarding the Seismic Hazard Analysis, it has been necessary to determine the probability curves for exceeding peak ground accelerations in the range [1÷500 years] by using the Poisson method shown in the following equation:

Where λ is the rate of exceeding the intensity measure IM=PGA in the period ΔT.

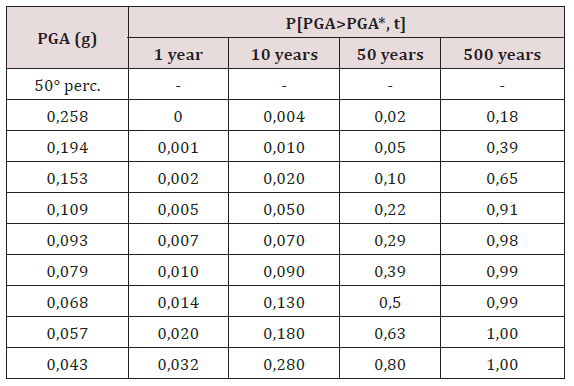
The probability of having PGA values greater than the PGA* ones, giving rise to the so-called exceedance probability curves (Figure 17). These curves show how the probability of overcoming a specific Intensity Measure (IM) in a number of year’s t follows a nonlinear law. In fact, with reference to an observation time t=500 years, the probability of exceeding PGA=0.05g is 100%, while it is equal to 19% for a PGA=0.25g. The Italian Institute of Geophysics and Volcanology (INGV) [36] provides the probabilities of exceedance of PGA values for different annual frequencies. The results obtained are summarized in Table 2.

The overcoming of seismic events in a predetermined region is modelled on the basis of the hypothesis of the Poissonian stochastic process [37]. The Poisson process is defined by the probability that the number of events occurred in the time interval [0, T=t], indicated by the random variable Xt, assumes a set of variables x. The probability of occurrence of X=x seismic events in the period T is expressed through the following equation:

Where ν is the annual frequency of events and T is the return period of the seismic event. With reference to the Poisson process, it has been possible to consider return periods (Tr) associated to the Limit States defined in the Italian code NTC08 [38] corresponding to the exceeding probabilities of 81%, 63%, 10% and 5%, respectively (Table 3).

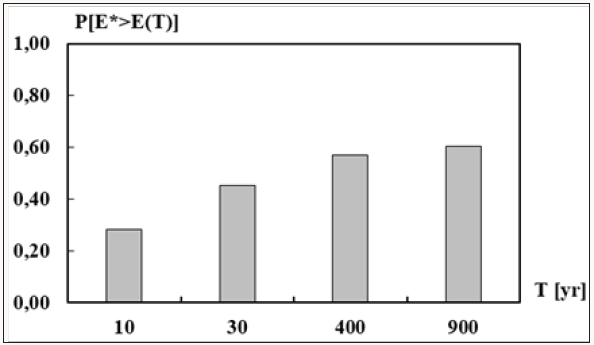
So, established the return period, the probability of having at least one seismic event in the time t is estimated. In particular, it has been possible to define time periods t of 10, 30, 400, 900 years, which have been associated to the probability of exceedance (Figure 18). The probability of having a seismic event increases with increasing number of years. In fact, considering T_r=30 years at a time t=10 years, the probability of occurrence (P[E>E*(10years)])=28%; while for T_r=975 years in t=900 years, the probability of occurrence is 60%. These considerations allowed estimating the seismic risk index (IRI) for the study sub-sector. The investigation goal has been the estimation of the occurrence probability of the seismic risk R_i, associated to the damage Di at the time t, as a function of both the magnitude Mi and the epicentral distance d=d_epi.

In particular, the risk index IRI evaluated for each damage level Di, by varying the magnitude Mi and the epicentral distance depi, has been calculated through the following relationship [38,39]:

In this case, it has been taken into account the cumulative damage associated with the different magnitudes included in the range [4÷6] within the considered interval of epicentral distances [5÷30 Km]. Finally, the sub-sector risk Ri can be interpreted as the average value of the risk indices IRI associated to each observation time t:

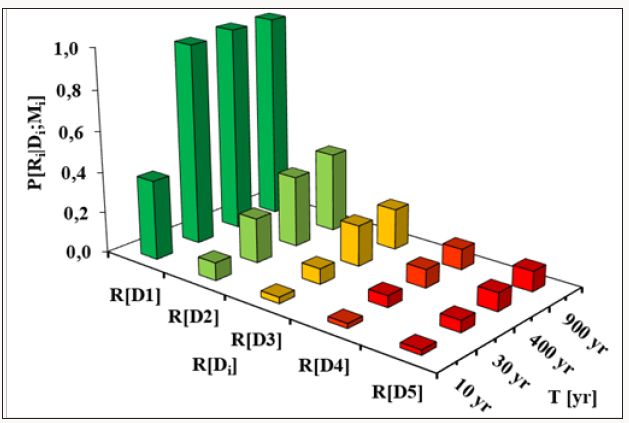
Figure 19 shows that the risk exceeding probability associated with the damage D1 in 10 years is 40% and it increases with the observation period up to attain 100% for t=900 years. Similarly, considering the risk associated to the damage threshold D5, it can be noted that it tends to increase from 0% for t=10 years to a maximum value of 10% referred to an observation period t=900 years.

Conclusion

The study illustrated has provided the seismic risk and damage assessment of an urban sector within the historical centre of Sant’Antimo by using a probabilistic scenario method. Firstly, with reference to the typological characterization analysis through the CARTIS form, the types of buildings within the sub-sector 1 of the inspected centre, as well as the volumes of the buildings detected there, have been identified so to individuate the built-up exposure to seismic events. In this case, being an urban-scale risk analysis, it has been assumed as a hypothesis to associate a greater exposure to larger built volumes. Subsequently, the seismic vulnerability analysis has been carried out by using an appropriate vulnerability form taking into account the aggregate conditions of individual structural units. Based on this survey tool, the expected damages for different seismic intensities have been evaluated through the derivation of fragility curves. From these curve, it has been obtained that for modest seismic intensities, buildings shows zero or very slight damage levels, whereas for high intensities almost all buildings should collapse.

Later on, a risk analysis has been conducted by taking into account the distribution of epicentre distances and magnitudes variable in the range [4÷6]. Applying the Crespellani’s seismic intensity attenuation law, through the variation of input parameters (magnitude and site-source distance), it has been possible to determine the expected maximum seismic intensities in order to estimate the associated degree of damage that the examined built up should exhibit under different grade earthquakes. The achieved results have shown that, for distances very close to the site of interest, the expected damage increases when seismic intensity increases. In fact, for R=5 Km and for a value of the expected seismic intensity ranging from 6 to 10, it can be noticed that the damage progressively increases in term of collapsed buildings in the study area. Moreover, the risk index associated with any degree of expected injury has been calculated. This index has allowed estimating the probability that a given event E at a time t may be able to produce a damage D in the sub-urban sector analysed.

From the analysis of results it has been possible to perceive that, with observation times ranging from 10 to 900 years, when the intensity of ground motion increases, the probability of exceeding the null damage threshold (D1) increases much more than the probability to attain the collapse limit (D5) of all the buildings placed in the area. In fact, it can be noted that, as an example, for t=10 years, the probability of having a risk associated with the damage D1 is 40%, while it is null for the risk associated to the damage D5. Conversely, for an observation period of 900 years, the probability of risk associated to the damage D1 is 100%, while this probability is equal to 10% for the damage level D5. This makes clear the conclusion that, in case of slow motions, buildings either do not suffer any damage or exhibit moderate damage, whereas in case of strong motions the risk associated to the structural collapse increases.

References

1. Cardona OD, Van Aalst MK, Birkmann J, Fordham M, McGregor G, et al. (2012) Determinants of risk: exposure and vulnerability. In: Managing the Risks of Extreme Events and Disasters to Advance Climate Change Adaptation. Cambridge University Press, Cambridge, UK, pp. 65-108.
2. Dolce M, Zuccaro G (2003) SAVE Project: Updated Tools for the Seismic Vulnerability Evaluation of the Italian Real Estate and of Urban Systems. 1st year report, National Group for Protection from earthquake (GNDT), Rome.
3. Dolce M, Goretti A (2015) Building damage assessment after the 2009 Abruzzi earthquake. Bulletin of Earthquake Engineering 13(8): 2241- 2264.
4. Coburn AW, Spence R (2002) Earthquake protection (2nd edn.); Wiley, Chichester, England.
5. Calvi GM, Pinho R, Magenes G (2006) Development of seismic vulnerability assessment methodologies over the past 30 years. ISETJ Earthq Technol 43(3): 75-104.
6. Benedetti D, Petrini V (1984) Sulla vulnerabilità si sismica di edifici in muratura: Un metodo di valutazione (in Italian). L Industria delle Costruzioni 149(18): 66-78.
7. Maio R, Ferreira TM, Vicente R, Estevao J (2015) Seismic vulnerability assessment of historical urban centre: case study of the old city centre of Faro, Portugal. Journal of Risk Research 19(5): 551-580.
8. National Group for Protection from earthquake GNDT (1993) Manuale per il rilevamento della vulnerabilità sismica degli edifici, istruzioni per la compilazione della scheda di 2° Livello (in Italian).
9. Lantada N, Irizarry J, Barbat AH, Goula X, Roca A, et al. (2010) Seismic hazard and risk scenarios for Barcelona, Spain, using the Risk-UE vulnerability index method. Bulletin of Earthquake Engineering 8(2): 201-229.
10. D Ayala DF, Paganoni S (2011) Assessment and analysis of damage in L’Aquila historic city centre after 6th April 2009. Bulletin of Earthquake Engineering 9(1): 81-104.
11. Armaş I (2012) Multi-criteria vulnerability analysis to earthquake hazard of Bucharest, Romania. Natural Hazards 63(2): 1129-1156.
12. Vicente R, Parodi S, Lagomarsino S, Varum H, Mendes Silva JAR (2011) Seismic vulnerability and risk assessment: case study of the historic city centre of Coimbra, Portugal. Bulletin of Earthquake Engineering 9(4): 1067-1096.
13. Ferreira TM, Vicente R, Mendes Silva JAR, Varum H, Costa A (2013) Seismic vulnerability assessment of historical urban centres: Case study of the old city centre in Seixal, Portugal. Bulletin of Earthquake Engineering, 11(5): 1753-1773.
14. Simões A, Bento R, Lagomarsino S, Lourenço PB (2016) Simplified evaluation of seismic vulnerability of early 20th century masonry buildings in Lisbon. 10th International Conference on Structural Analysis of Historical Constructions (SAHC 2016), Leuven, Belgium, pp. 1513- 1519.
15. Bondarabadi HA, Mendes N, Lourenço PB (2016) Empirical seismic vulnerability analysis for masonry buildings based on school building survey in Iran. Bull Earthquake Engineering 14(11): 3195-3229.
16. Uva G, Sanjust CA, Casolo S, Mezzina M (2016) ANTAEUS Project for the Regional Vulnerability Assessment of the Current Building Stock in Historical Centres. International Journal of Architectural Heritage 10(1): 20-43.
17. Ferreira TM, Maio R, Vicente R (2017) Analysis of the impact of large scale seismic retrofitting strategies through the application of a vulnerability-based approach on traditional masonry buildings. Earthquake Engineering and Engineering Vibration 16(2): 329-348.
18. Giovinazzi S, Lagomarsino S (2004) A macro-seismic model for the vulnerability assessment of buildings. In Proceedings of 13th WCEE. Vancouver, Canada. International Journal of Architectural Heritage, pp. 22-40.
19. Grünthal G, Musson R, Schwarz J, Stucchi M (1998) European macroseismic scale. Centre Européen de Géodynamique et de Séismologie, Luxembourg, pp.101.
20. Cornell CA (1968) Engineering Seismic Risk Analysis. Bulletin of the Seismological Society of Amaerica 58(5): 1583-1606.
21. Iervolino I, Fabbrocino G, Manfredi G (2004) Un Metodo per la Analisi di Rischio Sismico a Scala Territoriale. XI Italian Conference on Earthquake Engineering (ANIDIS), Genova, Italy, p. 10.
22. Bommer JJ (2002) Deterministic Vs Probbilistic seismic hazard assessment: An exaggerated and obstructuve dichotomy. Journal Earth Eng 6(001): 43-73.
23. Carreno ML, Cardona OD, Barbat AH (2007) Urban Seismic Risk Evaluation: A Holistic Approach. Natural Hazard 40(1): 137-172.
24. Zuccaro G, Della Bella M, Papa F (1999a) Caratterizzazione tipologico strutturali a scala nazionale. 9th National Conference ANIDIS, Lingegneria Sismica in Italia, Torino.
25. Formisano A, Mazzolani FM, Florio G, Landolfo R (2010) A quick methodology for seismic vulnerability assessment of historical masonry aggregates. In COST Action 26, Urban Habitat Constructions under Catastrophic Events, Final Conference. Naples, Italy, pp 577-582.
26. Formisano A, Florio G, Landolfo R, Mazzolani FM (2015) Numerical calibration of an easy method for seismic behaviour assessment on large scale of masonry building aggregates. Advances in Engineering Software 80: 116-138.
27. Formisano A, Chieffo N, Mosoarca M (2017) Seismic Vulnerability and Damage Speedy Estimation of an Urban Sector within the Mucipality of San Potito Sannitico (Caserta, Italy). The Open Civil Engineering Journal 12: 1106-1121.
28. Giovinazzi S, Lagomarsino S (2004) A macroseismic method for the vulnerability assessment of buildings. Proceedings of the 13th World Conference on Earthquake Engineering, Vancouver, Canada, pp. 896.
29. Giovinazzi S, Balbi A, Lagomarsino S (2004) A vulnerability model for buildings in historical centres (in Italian). Proceedings of the 12th Italian Conference on Earthquake Engineering (ANIDIS), Genoa, Italy.
30. Bernardini A, Giovinazzi S, Lagomarsino S, Parodi S (2007) Implicit Damage Probability Matrixes in the EMS-98 European Macroseismic Scale. Proceedings of the 12th Italian Conference on Earthquake Engineering (ANIDIS), Pisa, Italy.
31. Bernardini A, Giovinazzi S, Lagomarsino S, Parodi S (2007) Vulnerability and damage assessment at a territorial scale according to a macroseismic approach coherent with EMS-98 European Macroseismic Scale. Proceedings of the 12th Italian Conference on Earthquake Engineering (ANIDIS), Pisa, Italy.
32. UNDRO (1979) Natural Disasters and Vulnerability Analysis. Report of Expert Group Meeting.
33. Crespellani T, Madiai C (1991) Seismic hazard and site effects in the Florence area. X ECSMFE, p. 81-84.
34. Reiter L (1990) Earthquake hazard analysis: Issues and insights. Columbia University Press, New York, USA.
35. National Institute of Geophysics and Volcanology (INGV) Mappe Interattive di Pericolosità Sismica (in Italian).
36. Zuccaro G (2005) Inventario e vulnerabilità del patrimonio edilizio residenziale del territorio nazionale mappe di rischio e perdite socio economiche. GNDT, Roma.
37. Ministerial Decree of Public Works (NTC08) New Technical Codes for construction (in Italian). Official Gazette of the Italian Republic, Rome, Italy, pp. 1-438.
38. Cornell CA, Jalayer F, Hamburger RO, Foutch DA (2002) The Probabilistic Basis for the 2000 SAC/FEMA Steel Moment Frame Guidelines. Journal of Structural Engineering 128(4).
39. Formisano A, Chieffo N, Mosoarca M (2017) Probabilistic damage scenario: a case study in Amatrice affected by the 2016 seismic sequence. 3rd International Conference on Protection of Historical Constructions, Lisbon, Portugal.